Electron Shuttling Model

From MaRDI portal
Revision as of 10:22, 9 April 2025 by Shehu (talk | contribs)

User:Koprucki:Examples:myTest



quantum dynamical model of an electron to be shuttled in a silicon quBus device

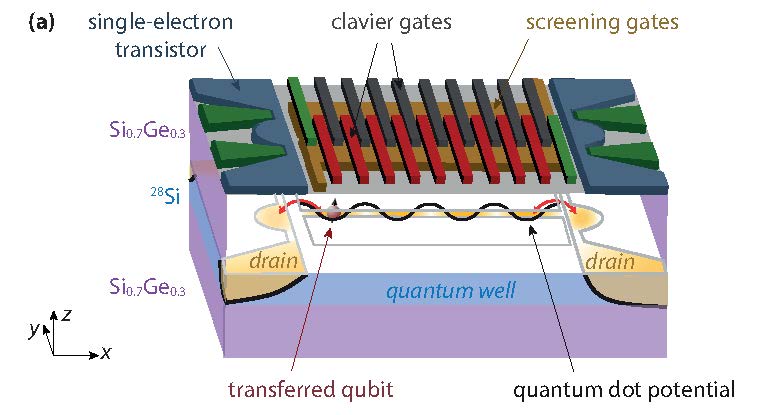
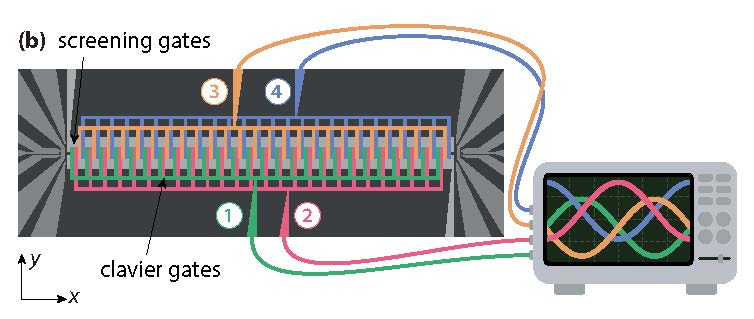
The clavier gate electrodes on the top surface generate a moving array of QD potentials
Top view on the Si-QuBus with the four different clavier gate sets highlighted in color




Error: No entity ID provided

The Electron Shuttling Model contains the following mathematical expressions with quantities:

Lua error in Module:TA4TK:myTest at line 272: attempt to index local 'alttext' (a nil value).

The Electron Shuttling Model is applied by the following computational tasks:

Optimal Control
Quantum Time Evolution
Quantum Stationary States
Semiconductor Charge Neutrality




Retrieved from "https://staging.mardi4nfdi.org/w/index.php?title=User:Koprucki:Examples:myTest&oldid=17947"
Powered by MediaWiki